5y2 X38 Y2+5x312 By Elimination Method
Step 1 Solve one of the equations for either x = or y = We will solve second equation for y Step 2 Substitute the solution from step 1 into the second equation Step 3 Solve this new equation Step 4 Solve for the second variable The solution is (x, y) = (10, 5) Note It does not matter which equation we choose first and which second.
5y2 x38 y2+5x312 by elimination method. Click here👆to get an answer to your question ️ Solve the following pair of equations 5y/2 x/3 = 8 y/2 5x/3 = 12. The intersection of the two graphs is ( 2;. 3x5y=2 Geometric figure Straight Line Slope = 10/00 = 0600 xintercept = 2/3 = yintercept = 2/5 = Rearrange Rearrange the equation by subtracting what is.
Formatted Problem 1 Solve Using an Inverse Matrix x2y=1 , 4x5y=13 x2y= 1 x 2 y = 1 , 4x5y= 13 4 x 5 y = 13 2 Multiply the Matrices 1/ ( square root of 17),4/ ( square root of 17) 1/ ( square root of 17), 4/ ( square root of 17) 1 √17 − 4 √17⎡. 1) So the solution to the system of simultaneous equations is x = 2 and y = 1 We can also check the solution using algebraic methods Substitute equation ( 1) into ( 2) x = 2 y ∴ y = 2 ( 2 y) − 3 Then solve for y y − 4 y = − 3 − 3 y = − 3 ∴ y = 1. Solve by elimination method 5xy=1 10x12y=2 Please Help I can't seem to figure this one out Algebra Solve the following systems of equations using the addition (elimination) method 5xy=2 3xy=1 Algebra Solve by elimination method 5xy=1 10x12y=2 Please Help I can't seem to figure this one out Algebra.
Substituting a = 6 in equation (1), We get 2(6) – b = 3 ⇒ 12 – b = 3 ⇒ b = 9 Thus, the two numbers are 6 and 9 respectively 4 The sum of two positive numbers x and y (x > y) is 50 and the difference of their squares is 7. Linear Equation in two variables is an important topic in the study of straight lines It comes under the section of Algebra in various government competitive examinations as well as in various entrance exams To solve linear equations in two variables, one must have strong basic knowledge of the concepts and methods involved. Consider a normal equation in #x# such as #3x=6# To solve this equation you simply take the #3# in front of #x# and put it, dividing, below the #6# on the right side of the equal sign #x=6/3=3^1*6=2# at this point you can "read" the solution as #x=2# With a system of #n# equations in #n# unknowns you do basically the same, the only difference is that you have more than 1 unknown.
Free simplify calculator simplify algebraic expressions stepbystep. X 2y 3z = 4 5x 6y 7z = 8 9x 10y 11z = 12 is equivalent to the vector equation x 2 4 1 5 9 3 5 y 2 4 2 6 10 3 5 z 2 4 3 7 11 3 5= 2 4 4 8 12 3 5;. Systems of equations 2 Solve the system 5 x − 3 y = 6 4 x − 5 y = 12 \begin {array} {l} {5x3y = 6} \\ {4x5y = 12} \end {array} 5x−3y = 6 4x−5y = 12 See answer › Exponential and logarithmic functions Solve for x 3 e 3 x ⋅ e − 2 x 5 = 2.
Steps for Solving Linear Equation x = 2 y 5 x = 2 y − 5 Swap sides so that all variable terms are on the left hand side Swap sides so that all variable terms are on the left hand side 2y5=x 2 y − 5 = x Add 5 to both sides Add 5 to both sides. NCERT Solutions Class 12 MicroEconomics;. 1 The modification of Gauss elimination method is called as ___________ a) Gauss Seidal b) Gauss Jordan c) Jacobi’s Method d) Relaxation Method Answer b Clarification The modified method of Gauss Elimination is called as Gauss Jordan method as it involves few changes in the procedure of Gauss Elimination 2.
This method of finding a solution to a system of equations is called Cramer’s rule (x r, y r);. Algebra I am not getting any of the posted answers Solve the system using elimination 2x 6y = 12 and 5x – 5y =10 (2, 1) (0, –2) (–2, 0) (1, 2) Algebra 2 Solve the system by elimination 2x 2y 3z = 0 2x y z =3. Linear Equations in Two Variables LINEAR EQUATIONS IN TWO VARIABLES An equation of the form ax by c = 0, where a, b, c are real numbers (a ≠0, b 0), is called a linear equation in two variables x and y Examples Each of the equations (i) 3x4y 2 = 0 (ii) 2x 5y = 9 (iii) OAx 03y = 27 (iv) 4lx J3y = 0 is a linear equation in x and y SOLUTION OF A LINEAR EQUATION.
Substitution method Given equations are 5x2y = 8 > (1) 2xy = 3 Subtract 2x from each side 2x2xy = 32xy = 2x3 Multiple to each side by negitive one y = 2x3 > (2) From (2) substitute the y value in (1) 5x2(2x3) = 8 5x4x6 = 8 x6 = 8 Subtract 6 from each side x66 = 86 x = 2 Substitute the x value in (1) 5(2)2y = 8 102y = 8 Subtract 10 from. For Solving Pair of Equation, in this Exercise Use the Method of Elimination by Equating Coefficients 5y/2 X/3 = 8 Y/2 5x/3 = 12 Mathematics Shaalaacom. For solving pair of equation, in this exercise use the method of elimination by equating coefficients 3 (x 5) = y 2 2 (x y) = 4 3y.
The elimination method of solving systems of equations is also called the addition method To solve a system of equations by elimination we transform the system such that one variable "cancels out" Example 1 Solve the system of equations by elimination $$ \begin{aligned} 3x y &= 5 \\ x y &= 3 \end{aligned} $$. If x is 5, we could use the second equation right over here We get y plus 2 times 5 I'll do it in that same color 2 times 5 is equal to 13 Or y plus 10 is equal to 13 We can subtract 10 from both sides And we will get y These cancel out y is equal to 3 So our solution that we get for x and y is that x is equal to 5 And y is equal to 3. Subtract 2 from 3 5x −5y = − 15 5x 3y = 1 0 − 8y = −16 Divide by −8 y = −16 −8 = 2 Substitute this is 1 x − (2) = −3 Add 2 to both sides.
Solve the following system of equations using elimination 5xy=24 ;. By applying the value of y and z in (1), we get x 2(4) 4 = 3 x 8 4 = 3 x 4 = 3 x = 1 So, the solution is (1, 4, 4) Question 2 Solve the following systems of linear equations by Gaussian elimination method 2x 4y 6z = 22, 3x 8y 5z = 27, − x y 2z = 2 Solution. Graph 2x5y=8 2x 5y = 8 2 x 5 y = 8 Solve for y y Tap for more steps Subtract 2 x 2 x from both sides of the equation 5 y = 8 − 2 x 5 y = 8 2 x Divide each term by 5 5 and simplify Tap for more steps Divide each term in 5 y = 8 − 2 x 5 y = 8 2 x by 5 5.
Example 28 Solve the following system of equations by matrix method 3x – 2y 3z = 8 2x y – z = 1 4x – 3y 2z = 4 The system of equation is 3x – 2y 3z = 8 2x y – z = 1 4x – 3y 2z = 4 Writing equation as AX = B 8(3&−2&3@2&1&−1@4&−3&2) 8(𝑥@𝑦@𝑧) = 8(8@1@4) H. Xy=3 Solutions for any system of equations can be found using the. NCERT Solutions Class 12 Accountancy Part 2;.
In this free online math video lesson on Solving Systems of Equations by Elimination we solve each system by elimination We solve 3x2y=2 and 5x5y=10 by el. System of Equations Solve with Elimination Method 1 x3y=5, x3y=1 2 xy=9, xy=3 3 2x8y=22, 8x8y=8 4 Get the answers you need, now!. The function applied to the zero value is always equal to 1 f (0) = a ^ 0 = 1 2 The exponential function of 1 is always equal to the base f (1) = a ^ 1 = a 3 The exponential function of a total is equal to the product of the use of the function on each value separately f (m n) = a ^ (m n) = a ^ m a ^ n = f (m) f (n).
System of equations step by step solver A system of equations is a collection of two or more equations with a same set of unknowns In solving a system of equations, we try to find values for each of the unknowns that will satisfy every equation in the system The equations in the system can be linear or nonlinear. Solving systems of linear equations using Gauss Seidel method calculator Solve simultaneous equations 2xyz=5,3x5y2z=15,2xy4z=8 using Gauss Seidel method, stepbystep online We use cookies to improve your experience on our site and to show you relevant advertising By browsing this website, you agree to our use of cookies. R = 1, 2, 3 i s D = 1 2 ∣ x 1 y 1 1 x 2 y 2 1 x 3 y 3 1.
Xy=5;x2y=7 Try it now Enter your equations separated by a comma in the box, and press Calculate!. Weekly Subscription $249 USD per week until cancelled Monthly Subscription $799 USD per month until cancelled Annual Subscription $3499 USD per year until cancelled. Problem 15 $\begin{array}{l} \frac{5x3y}{4} = \frac{x5y}{3} \\ 7x y = 12\end{array}$ Answer x = 2, y = 2 Problem 16 $\begin{array}{l} \frac{3x1}{5}2y3 = 0.
Answer 2x y = 3 6x 3y = 9 (equation 1) 7x 3y = 4 (equation 2) Adding equations (1) and (2) 13x = 13 x = 1 Substitute x = 1 into (1) 6(1) 3y = 9 6 3y = 9 y = 1 (x, y) = (1, 1). 5x4y=56 Solve for X/Y Please help me?. Algebra Calculator is a calculator that gives stepbystep help on algebra problems See More Examples » x3=5 1/3 1/4 y=x^21 Disclaimer This calculator is not perfect Please use at your own risk, and please alert us if something isn't working Thank you.
Use elimination to solve for x and y And they gave us two equations here x plus 2y is equal to 6 and 4x minus 2y is equal to 14 So to solve by elimination, what we do is we're going to add these two equations together so that one of the two variables essentially gets. Xyz=6 In addition this online equations solver can be used on any 2d system of equations (nonelinear system) eg 2xyy=9;. Or click the example About Elimination Use elimination when you are solving a system of equations and you can quickly eliminate one variable by adding or.
Answer (1 of 4) 2x5y1=0 (1) 3x7y=1 (2) Multiply eq (1) by 3 and eq (2) by 2 as 3*(2x5y1)=2*0 6x15y3=0 (3) 2*(3x7y)=2*1 6x14y=2 (4) Now subtract equation (4) from (3) as (6x15y3)(6x14y)=02 6x15y36x14y=2 y3=2 y=23 y=5 (5) Put the value of y from (5) into (1) as 2x5y1=0 2x. Download free PDF of best NCERT Solutions , Class 10, Math, CBSE Linear Equations in two variables All NCERT textbook questions have been solved by our expert teachers You can also get free sample papers, Notes, Important Questions. Click here👆to get an answer to your question ️ Solve the following pairs of linear equation by the elimination method and the substitution method(i) 3x 5y 4 = 0 and 9x = 2y 7 (ii) x2 2y3 =.
Therefore, x = –8 and y = –4 2 (i) ¾ x – 2/3 y = 1 3/8 x – 1/6 y = 1 (ii) 2x – 3y – 3 = 0 2x/3 4y ½ = 0 Solution (i) ¾ x – 2/3 y = 1 3/8 x – 1/6 y = 1 We can write it as ¾ x – 2/3 y = 1 (9x – 8y)/12 = 1 By cross multiplication 9x – 8y = 12. X/3 – y/12 = 4¾ 1/3(x y/4) = 19/4 1/3{(1 – 2y)/5 – (y/4)} = 19/4 (480 – 8y – 5y)/ = 57/4 (480 – 13y)/ = 57/4 480 – 13y = 285 13y = 195 y = 15 Now, on substituting the value of y in (iii), we get x = (1 – 2 × 15)/5 = (1 – 30)/5 = 90/5 = 18 Hence, the. Transcript Ex 34, 1 (Elimination) Solve the following pair of linear equations by the elimination method and the substitution method (i) x y = 5 and 2x – 3y = 4 x y = 5 2x – 3y = 4 Multiplying equation (1) by 2 2(x y) = 2 × 5 2x 2y = 10 Solving (3) and (2) by Elimination –5y = –6 5y = 6 y = 𝟔/𝟓 Putting y = 6/5 in (1) x y = 5 x 6/5 = 5 x = 5 – 6/5 x = (5 × 5.
X = 1/2, y = 1/3 Hence the solution is (1/2, 1/3) Question 2 Akshaya has 2 rupee coins and 5 rupee coins in her purse If in all she has 80 coins totalling ₹ 2, how many coins of each kind does she have Solution Let "x" and "y" number of 2 rupee and 5. Solve the following systems of simultaneous linear equations by the method of elimination by equating the coefficient 2x y = 13, 5x – 3y = 16 asked in Linear Equations in Two Variables by HarshKumar ( 327k points). The web calculator for simultaneous equations is free and easy to use It can also be used to solve a system of 3 unknowns such as 2xyz=9,4x5y8z=7;.
In that they have the same solution sets, namely, S = f( 2 z;3 2z;z) z 2Rg 24/323.
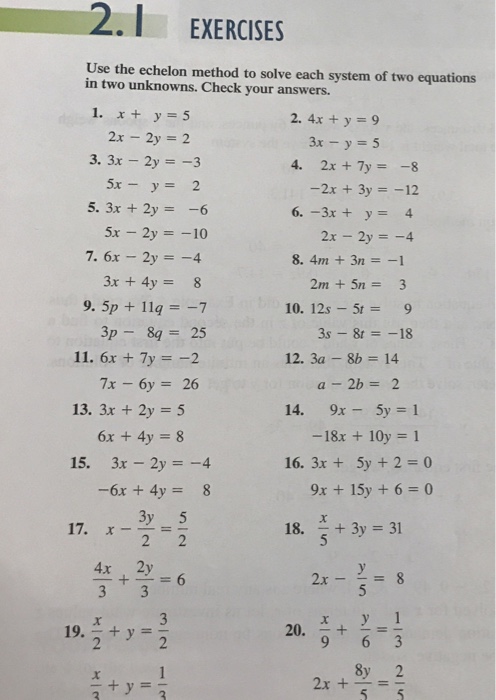
無料ダウンロード 3x 2y5 X5 Y

Ml Aggarwal Solutions For Class 9 Maths Chapter 5 Simultaneous Linear Equations
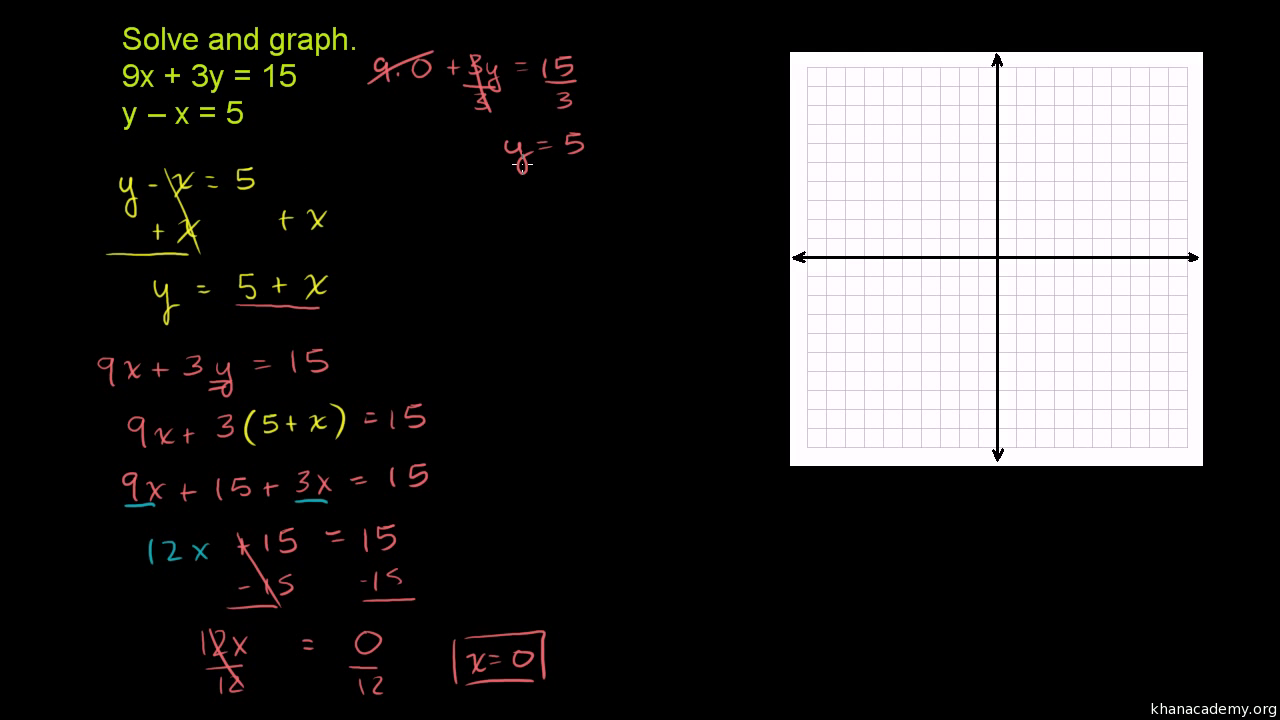
Systems Of Equations With Substitution 9x 3y 15 Y X 5 Video Khan Academy
5y2 X38 Y2+5x312 By Elimination Method のギャラリー

70以上 X5 Y5 Z5 ニスヌーピー 壁紙

Solving Systems Of Linear Equations Using The Elimination Method
Solve The Following Pairs Of Linear Equations By The Substitution Method 3x 2 5y 3 2 X 3 Y 2 13 6 Sarthaks Econnect Largest Online Education Community
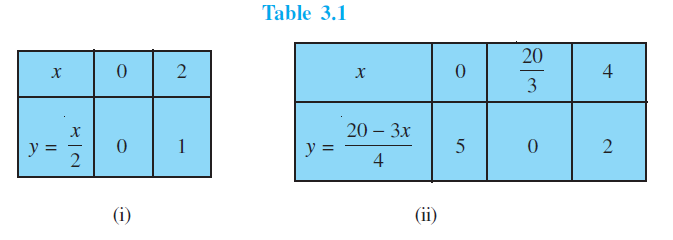
Pair Of Linear Equations In Two Variables Ncert Class 10 Maths
Sfdr Cisd Org
Home Bi No
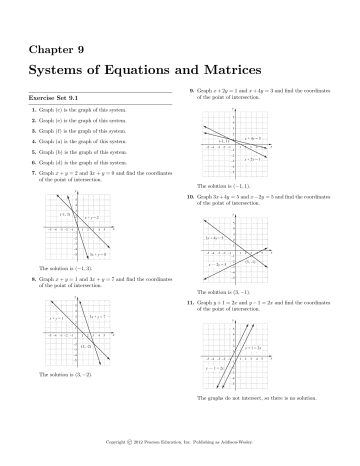
Chapter 9 Systems Of Equations And Matrices Manualzz

1 A Employ The Method Of Gaussian Elimination To Solve The System Of Linear Equations X 2y Homeworklib

Solve X Y 7 And 3x 2y 11

For Solving Each Pair Of Equations In This Exercise Use The Method Of Elimination By Equation Coefficiients 5y 2 X 3 8 Y 2 5x 3 12

Simultaneous Equations Pdf
Arithmetic And Algebra Worksheets Circle Manualzz

Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy

Solve The Following System Of Eq Using Elimination Method 1 5x 1 6y 12 And 1 3x 3 7y 8 Mathematics Topperlearning Com Tejj3bb

Expert Answer 3x 2 5y 3 2 X 3 Y 2 13 6 In Substitution Method Brainly In

無料ダウンロード 3x 2y5 X5 Y

1 A Employ The Method Of Gaussian Elimination To Solve The System Of Linear Equations X 2y Homeworklib
C H A P T E R 7 Manualzz
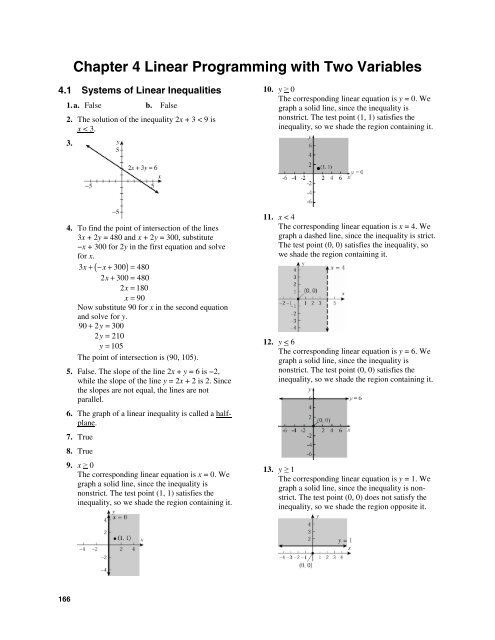
Chapter 4 Solutions Lake Central High School
Selected Answers Analytic Math Pdf Arab High School
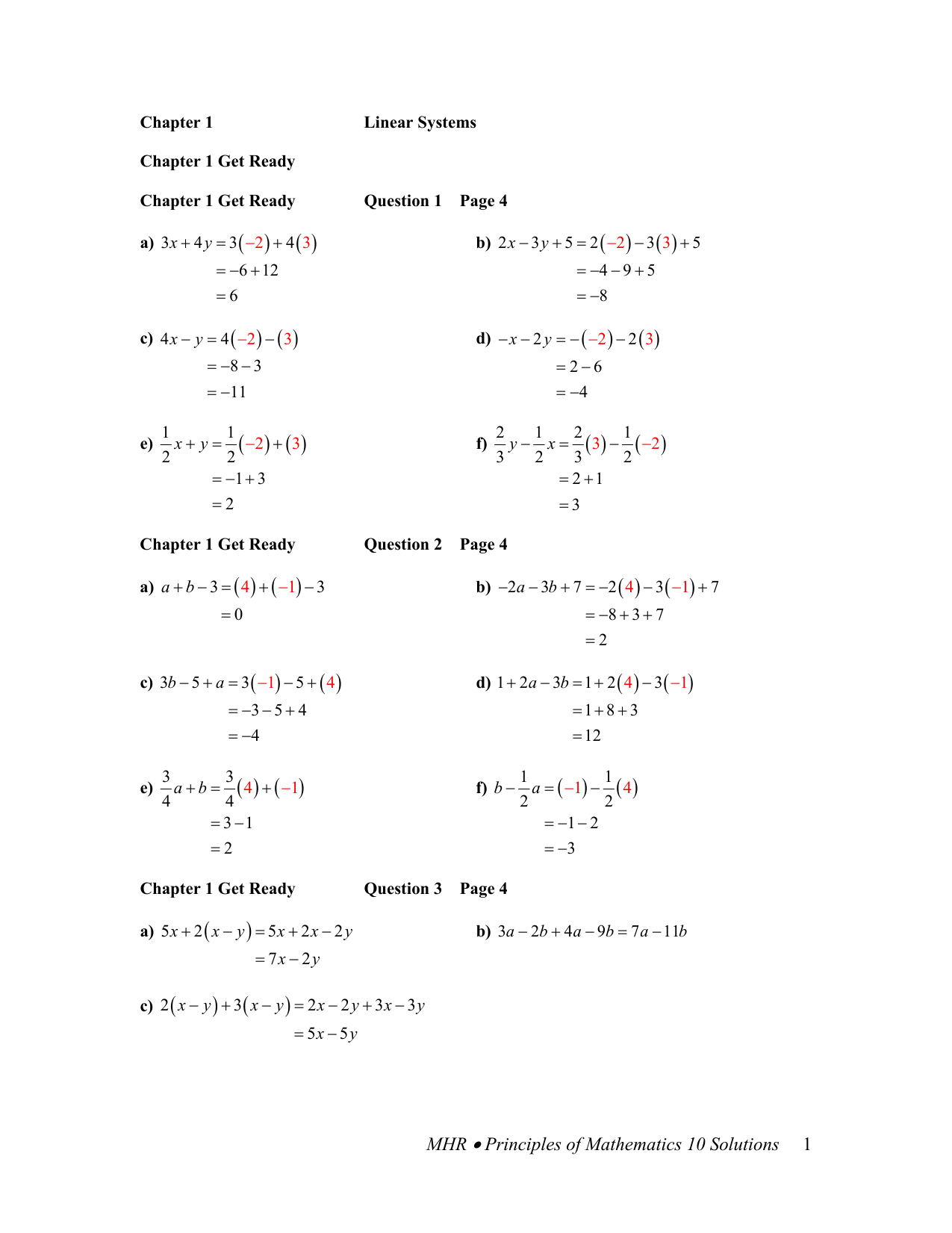
Mhr Principles Of Mathematics 10 Chapter 1
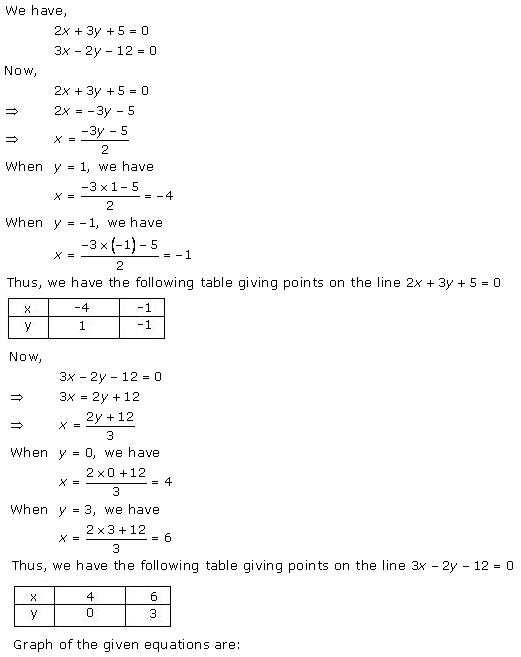
Rd Sharma Solutions For Class 10 Mathematics Cbse Chapter 3 Pairs Of Linear Equations In Two Variables Topperlearning

Solving Linear Systems
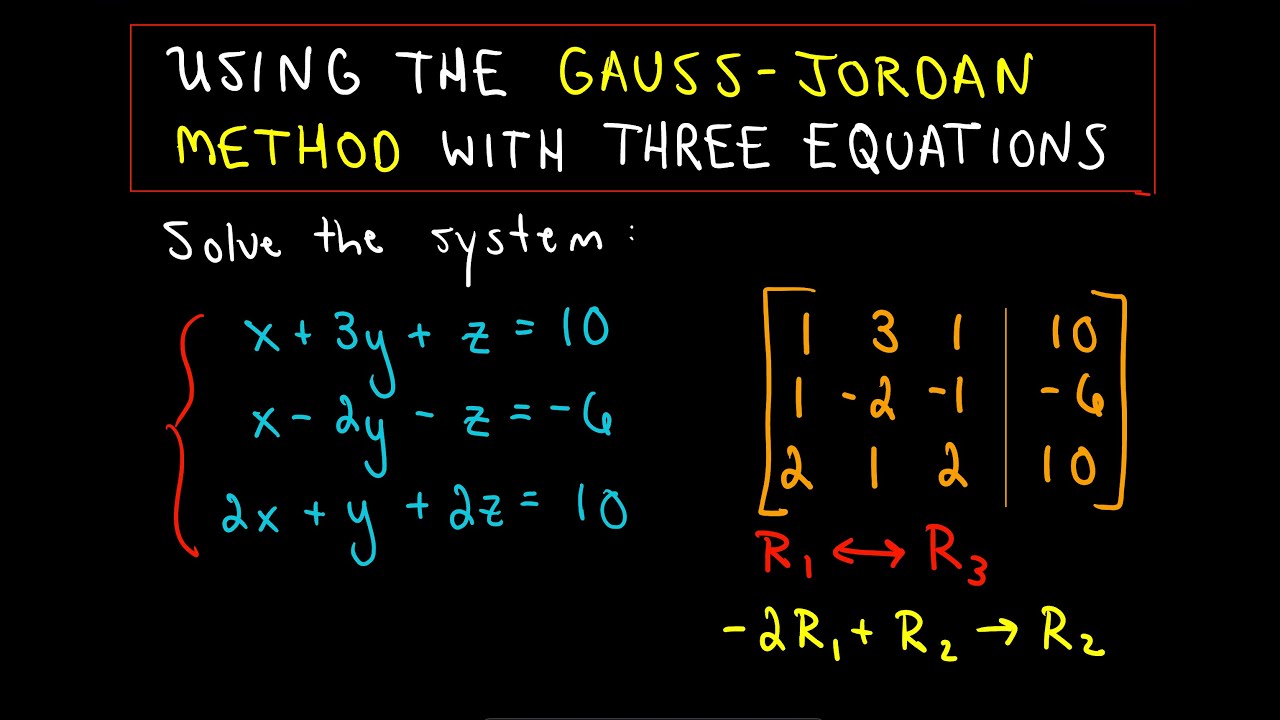
Using Gauss Jordan To Solve A System Of Three Linear Equations Example 1 Youtube

Algebra 1 Final Exam Review 5 Days 2nd Semester Ppt Download

Algebra 1 G9 Flip Ebook Pages 151 193 Anyflip Anyflip
Sfdr Cisd Org
1
Cbse 10 Math Cbse Linear Equations In Two Variables Ncert Solutions

Cramer S Rule To Solve A System Of 3 Linear Equations Example 2 Youtube

Cambridge International Examinations Cie Advanced Level Mathematics Pure Mathematics 2 3 By Ayman Alam Issuu
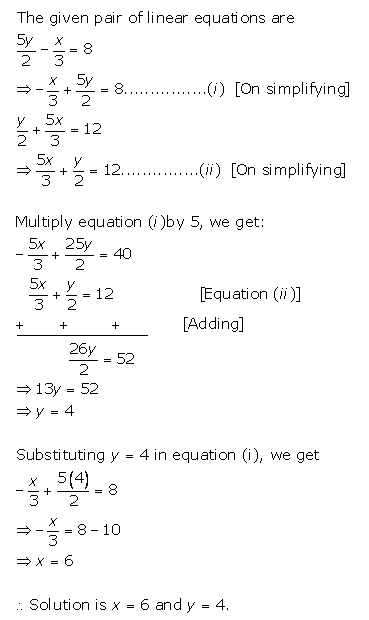
Please Solve This Simultaneous 5y 2 X 3 8 Y 2 5x 3 12 Mathematics Topperlearning Com Epa6spkk
Crispyteacher Weebly Com
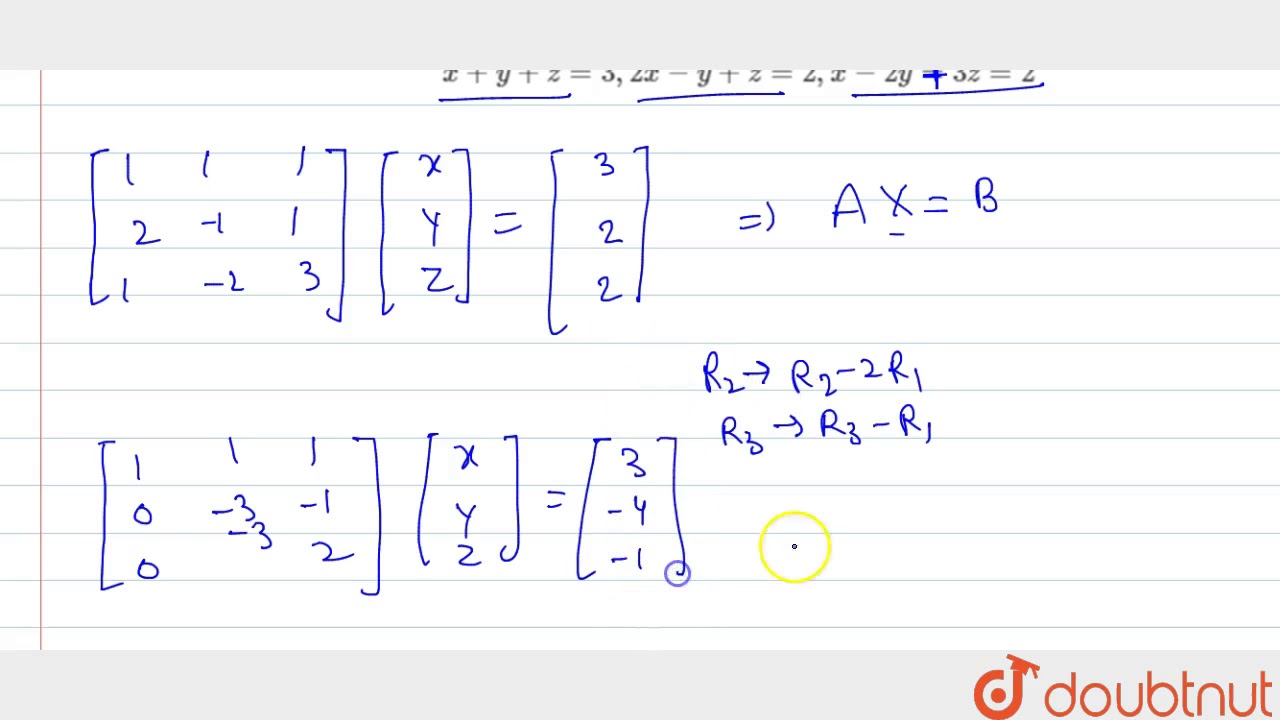
Solve The Following System Of Linear Equations By Matrix Method X Y Z 3 2x Y Z 2 X 2y 3z 2 Youtube

Linear Equations Questions And Answers Topperlearning

Graph Graph Inequalities With Step By Step Math Problem Solver
Solve The Following Pairs Of Linear Equations By The Substitution Method 3x 2 5y 3 2 X 3 Y 2 13 6 Sarthaks Econnect Largest Online Education Community

Maths Part One Ix Pages 51 100 Flip Pdf Download Fliphtml5
Shaalaa Com

If 3x 2y 24 And 2x 3y 18 Then Find X Y And X Y
Solve The Following Systems Of Linear Equations By Gaussian Elimination Method I 2x 2y 3z 2 X 2y Z 3 3x Y 2z 1 Sarthaks Econnect Largest Online Education Community
70以上 X5 Y5 Z5 ニスヌーピー 壁紙

5y 2 X 3 8and Y 2 5x 3 12
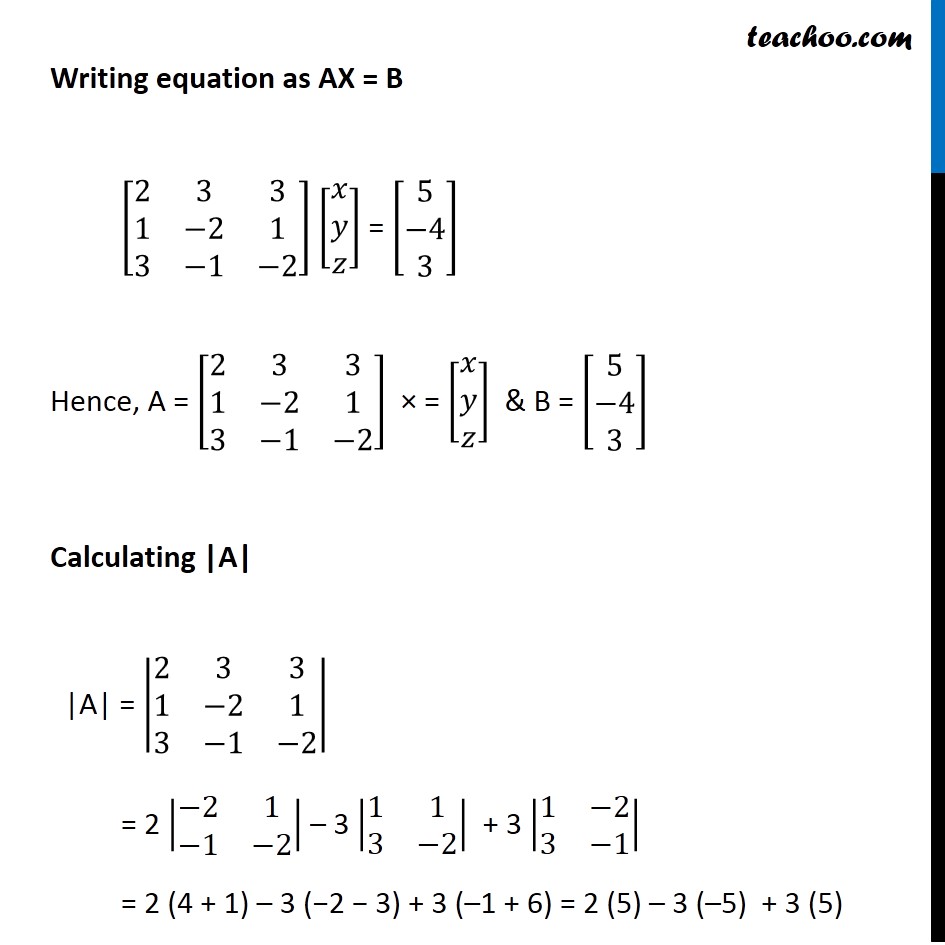
Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6

Rd Sharma Solutions For Class 10 Mathematics Cbse Chapter 3 Pairs Of Linear Equations In Two Variables Topperlearning
Cbse 10 Math Cbse Linear Equations In Two Variables Ncert Solutions
Solve The Following Equations By Cramer S Method I 7x 3y 15 12y 5x 39 Sarthaks Econnect Largest Online Education Community
Ssc Mathematics Chapter 4 Pair Of Linear Equations In Two Variables

最も好ましい X Y 2 4x 7y 30 2270 X Y 2 4x 7y 30 Josspixyr2a

Graphing Systems Of Equations Ppt Download

5y 2 X 3 8 Y 2 5x 3 12 Use Method Of Elimination Plz Do It Fast Plz Brainly In
How To Solve The System Of Equations Math Begin Cases X 2y 3z 7 2x Y Z 4 3x 2y 2z 10 End Cases Math Quora

2x 3 Y 3z 5 X 2y Z 4 3x Y 2z 3 Youtube

3 Systems Of Linear Equations
Cbse 10 Math Cbse Linear Equations In Two Variables Ncert Solutions
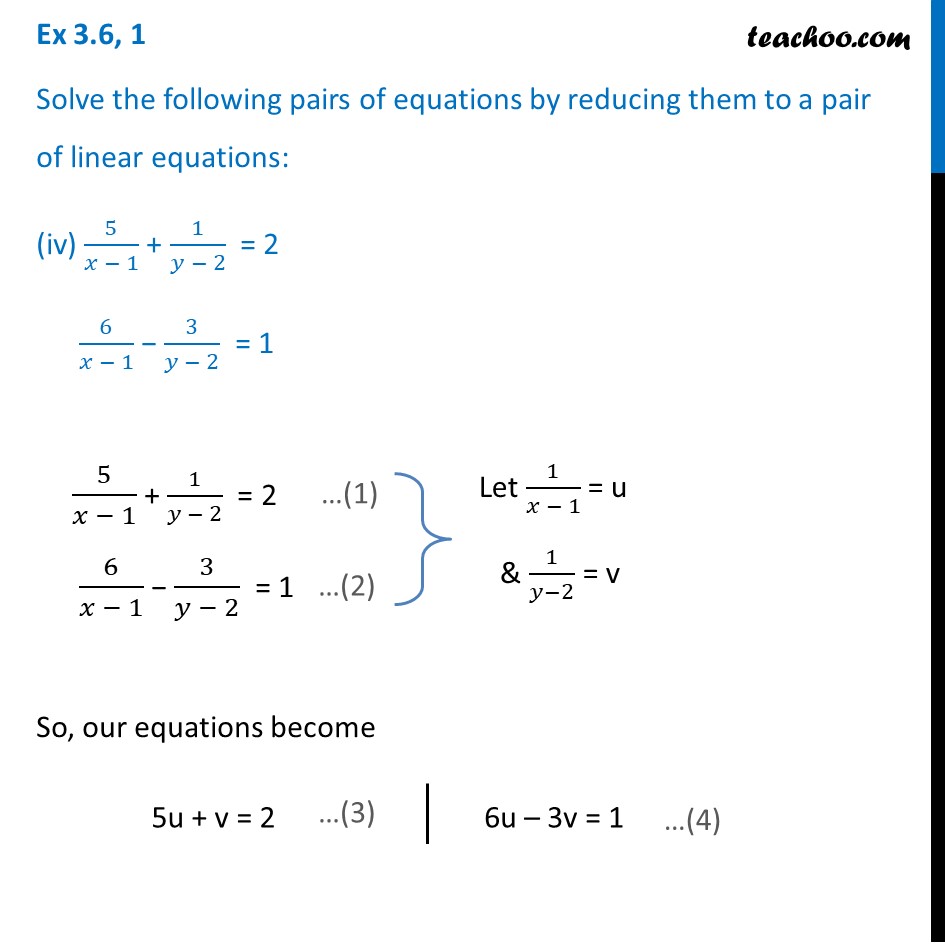
Ex 3 6 1 Iii Iv 4 X 3y 14 3 X 4y 23 Ex 3 6
Selina Chapter 6 Linear Equations Including Problems Icse Solutions Class 9 Maths

4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula
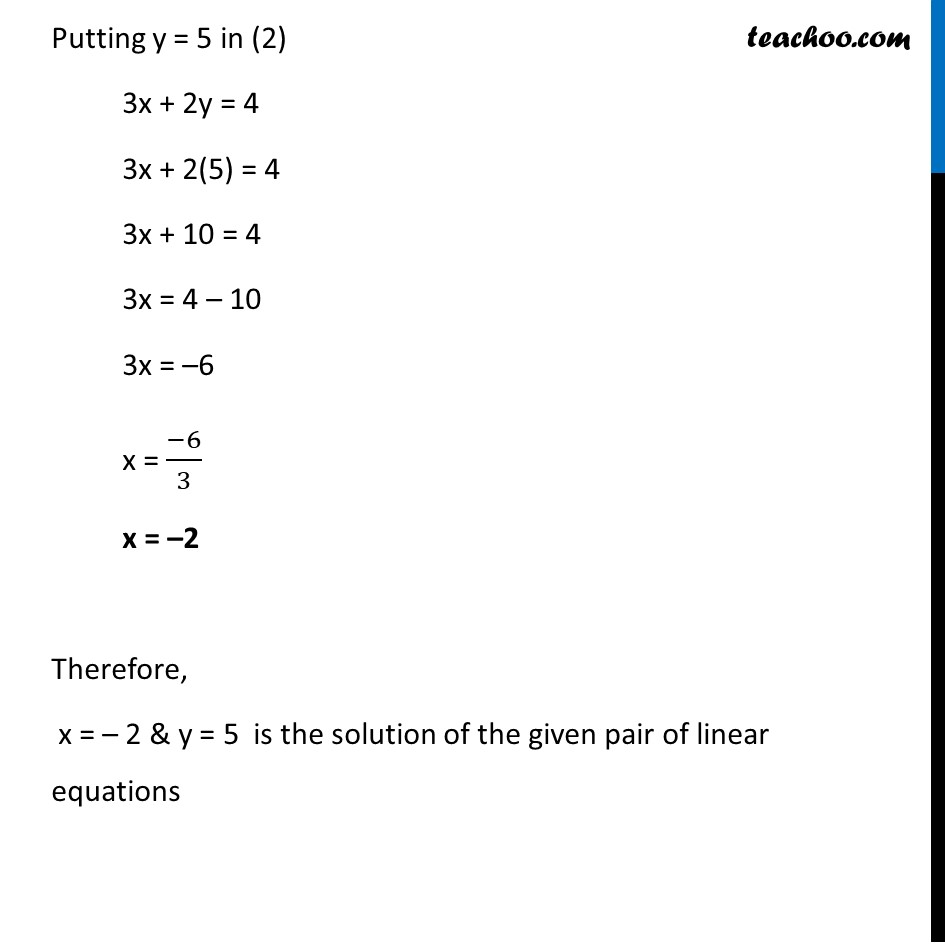
Ex 3 5 3 Solve By Substitution And Cross Multiplication Teachoo

Solve The System Of Linear Equation By Gauss Elimination Method 2x 3y Z 5 4x 4y 3z 3 2x 3y 2z 2 Brainly In

Solve For X Y X Y 8 2 X 2y 14 3 3x Y 12 11 Class 10 Cbse Sample Paper 17 18 Maths Brainly In

Chapter 3 System Of Linear Equations 3 1 Linear Equations In Two Variables Forms Of Linear Equation Forms Of Linear Equation Ax By C A B C Are Ppt Download
Chapter 5 Simultaneous Linear Equations Ml Aggarwal Icse Solutions For Class 9 Maths

4 4 Solving Simultaneous Equations Equations And Inequalities Siyavula

無料ダウンロード 3x 2y5 X5 Y
Substitution

Finite Mathematics 11th Edition Lial Solutions Manual By Vodomo Issuu
Mhr Principles Of Mathematics 10 Chapter 1

5y 2 X 3 8and Y 2 5x 3 12

70以上 X5 Y5 Z5 ニスヌーピー 壁紙

Simultaneous Equations Pdf
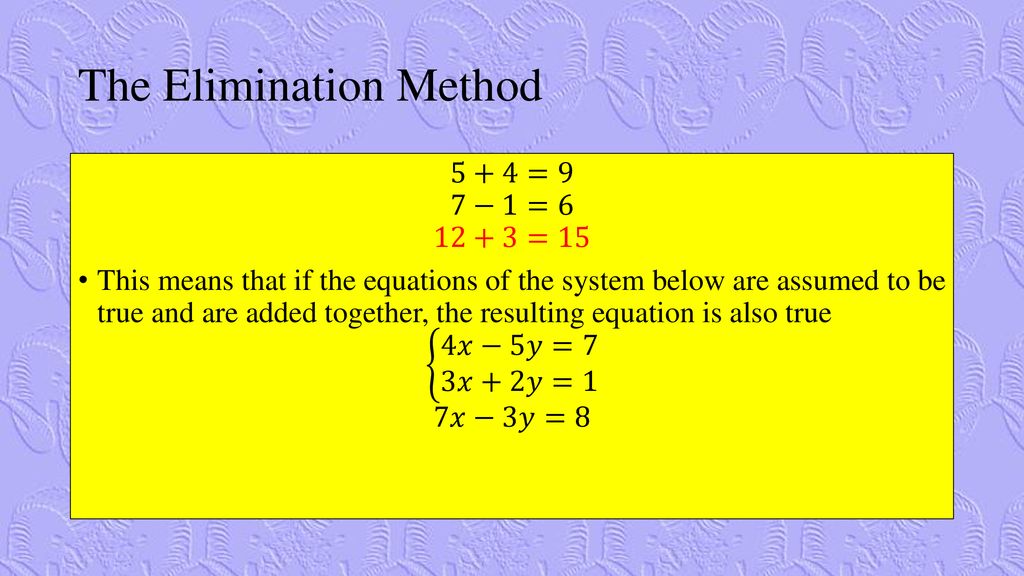
Solve Systems Of Linear Equations By Elimination Ppt Download

Finite Mathematics 11th Edition Lial Solutions Manual By Vodomo Issuu
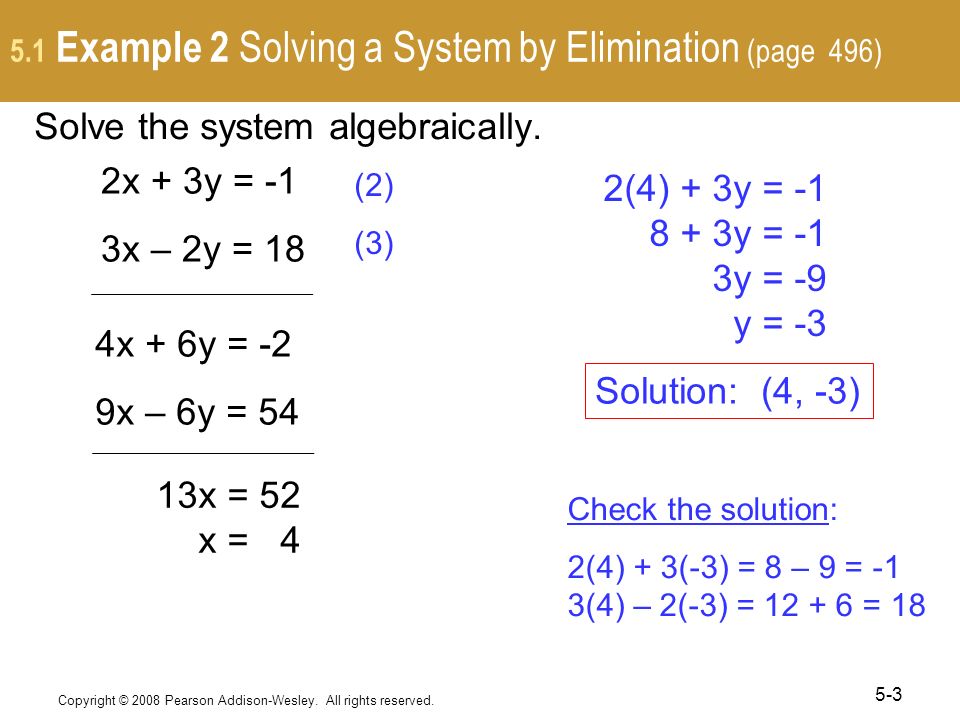
Copyright C 08 Pearson Addison Wesley All Rights Reserved 5 1 Systems Of Linear Equations 5 1 Linear Systems Substitution Method Elimination Method Ppt Download
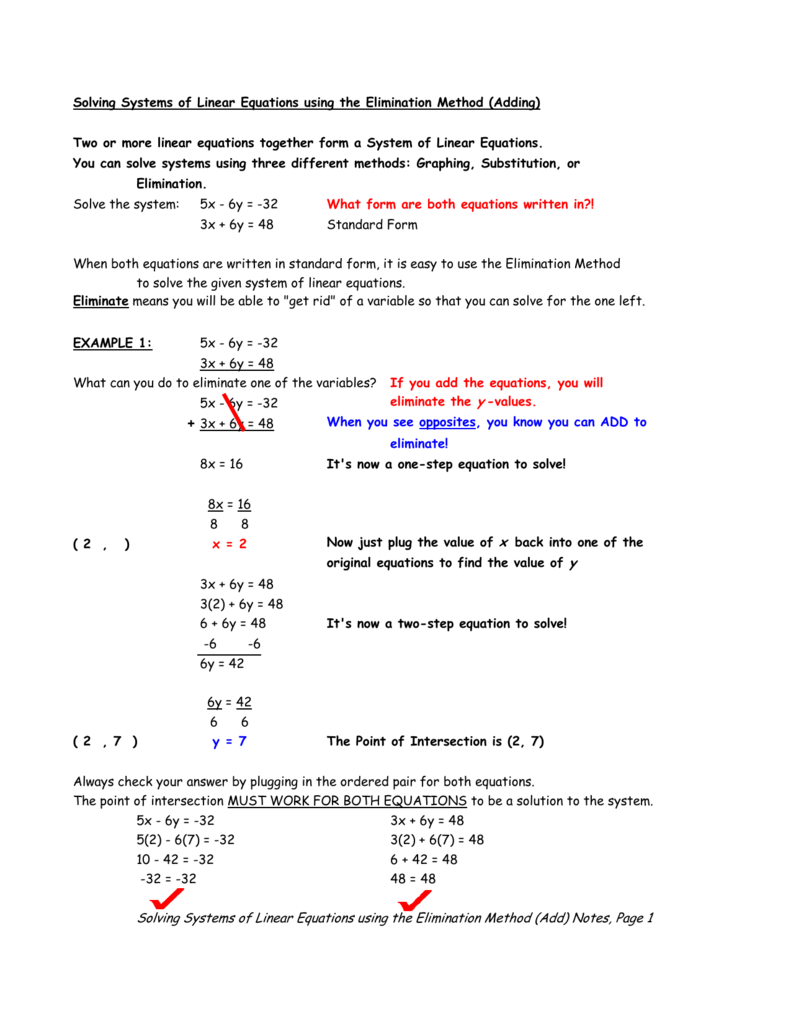
Solving Systems Of Linear Equations Using The Elimination Method
3 Systems Of Linear Equations

Solving Linear Systems

Solve The Following System Of Equation By Substitution Method X Y 7 3x 2y 11

R K Bansal Solutions For Class 8 Mathematics Maharashtra Chapter 6 Simultaneous Linear Equations Including Problems Topperlearning

If X Y 8 2 X 2y 14 3 3x Y 4 Then The Values Of X And Y Is
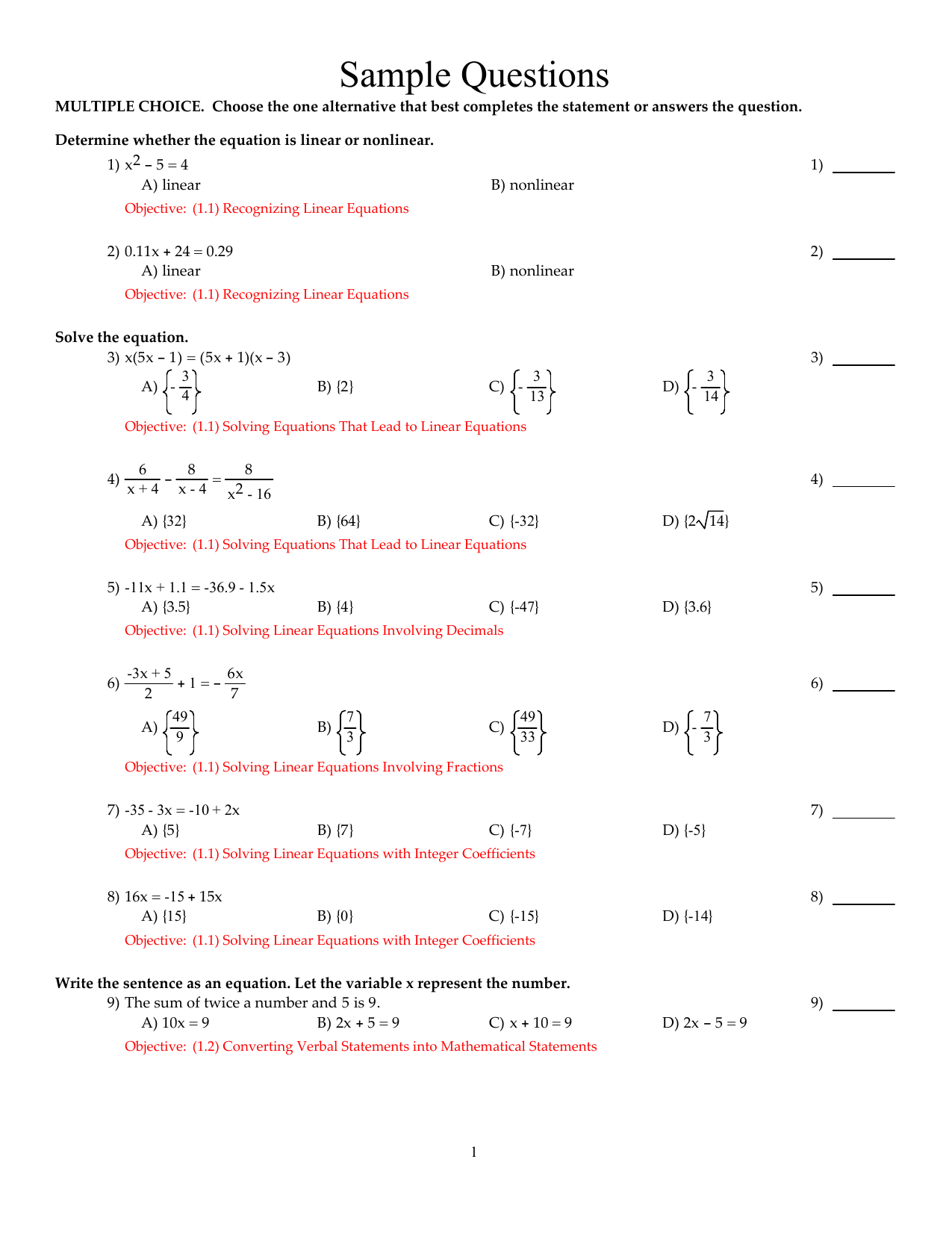
Test Sample Questions Manualzz
Elimination Method

5y 2 X 3 8 Y 2 5x 3 12 Use Method Of Elimination Plz Do It Fast Plz Brainly In
Selina Chapter 6 Linear Equations Including Problems Icse Solutions Class 9 Maths
1 1 Solving Real Life Problems 1 2 Multiplication And Division With Manualzz

5y 2 X 3 8and Y 2 5x 3 12
How To Solve This In Elimination 2x 5y 1 0 And 3x 7y 1 Quora

Maths Part 1 Eng Pages 51 100 Flip Pdf Download Fliphtml5
Waynesville K12 Mo Us
Selina Chapter 6 Linear Equations Including Problems Icse Solutions Class 9 Maths
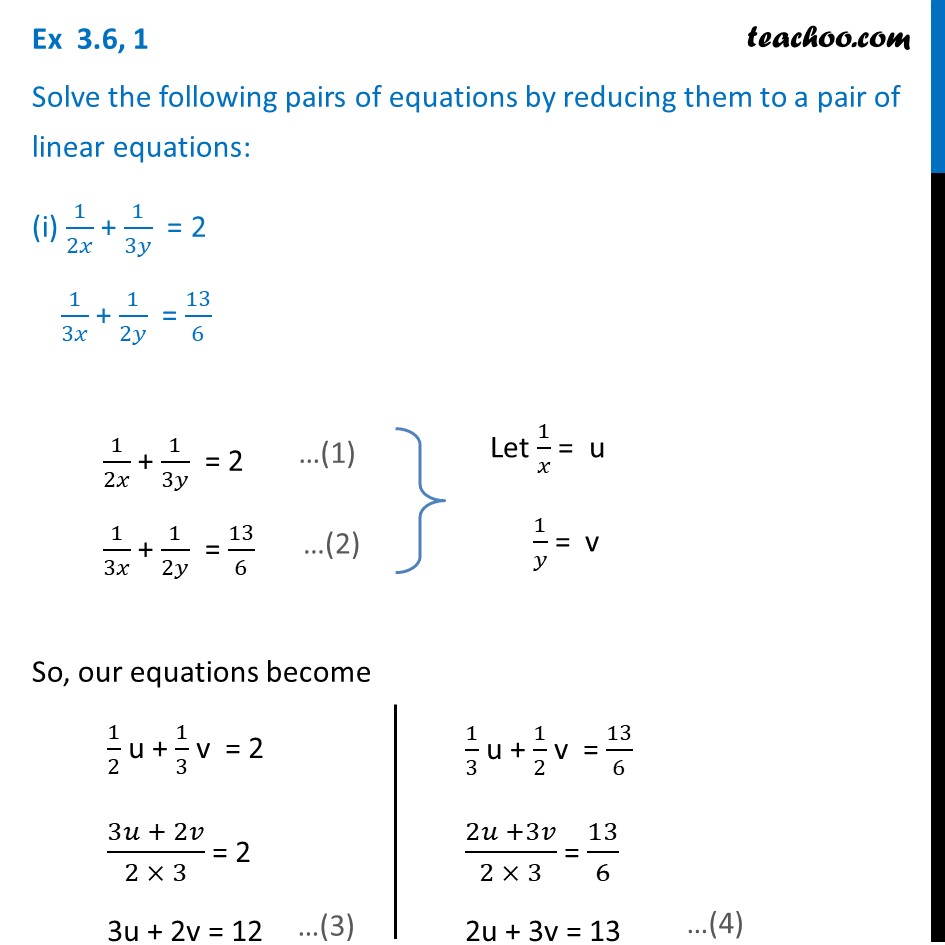
Ex 3 6 1 I And Ii Solve 1 2x 1 3y 2 1 3x 1 2y
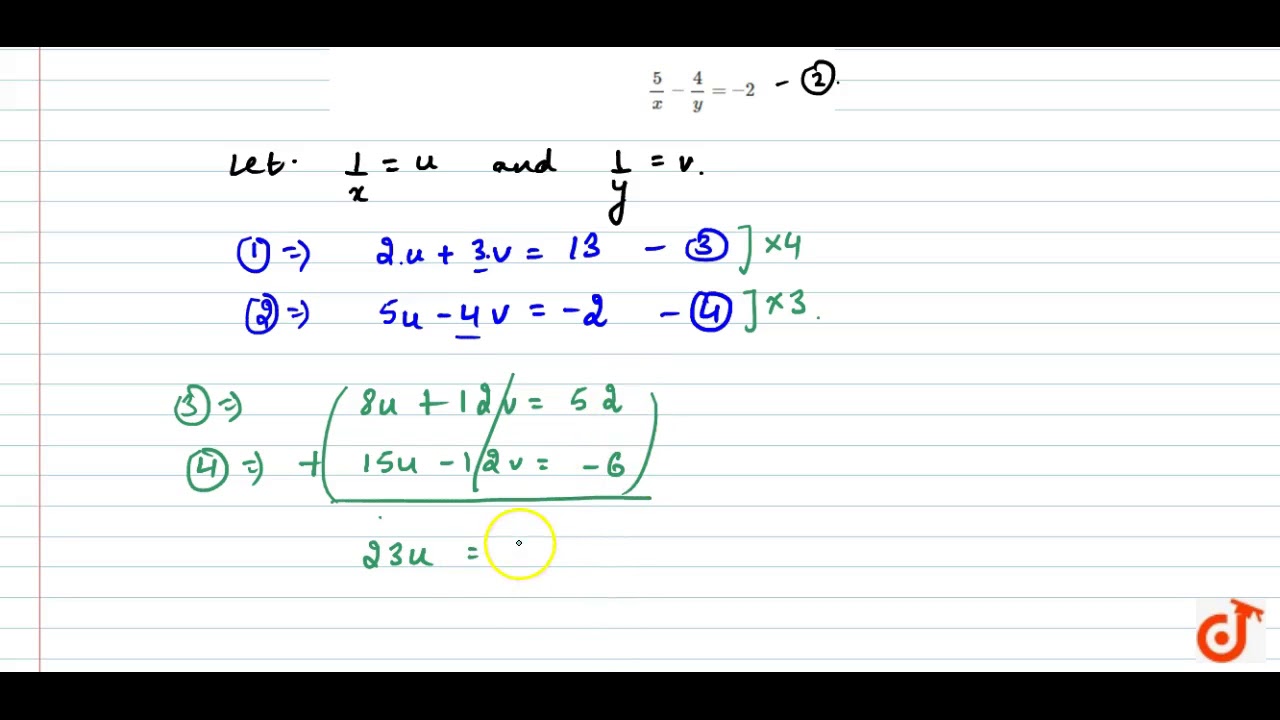
Solve The Pair Of Equations 2 X 3 Y 13 5 X 4 Y 2 Youtube

Algebra Connections Textbook Answers
Sfdr Cisd Org
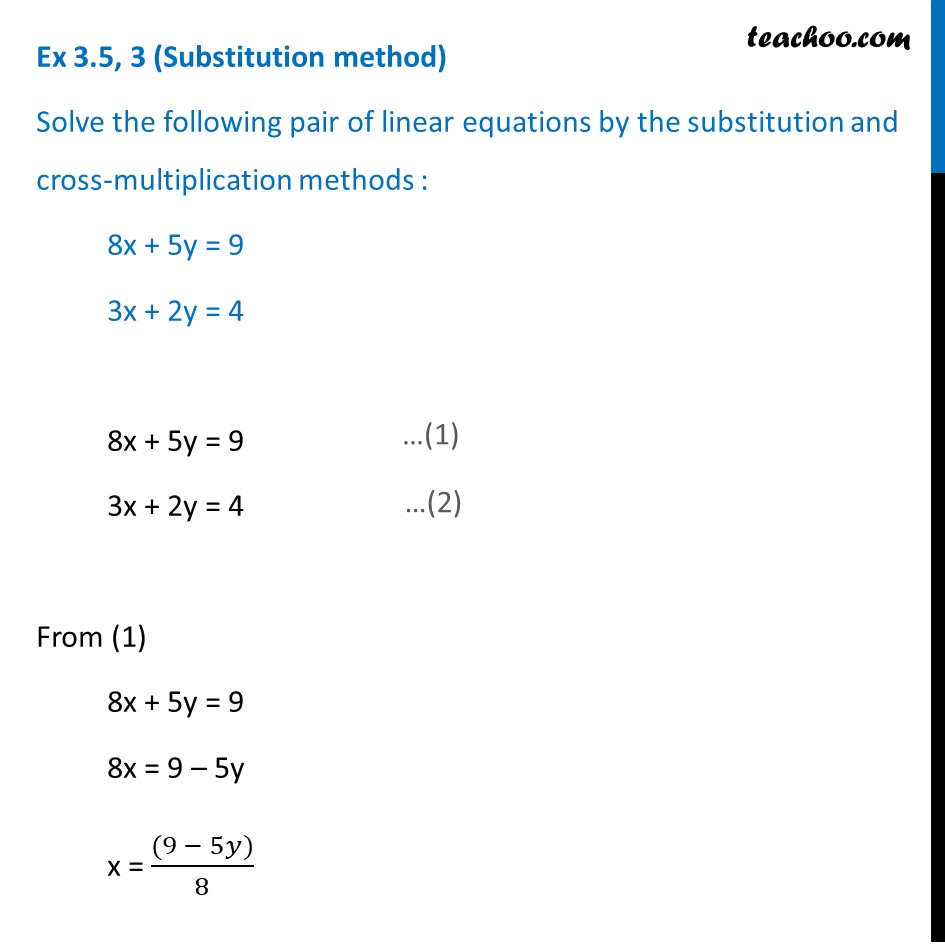
Ex 3 5 3 Solve By Substitution And Cross Multiplication Teachoo
1
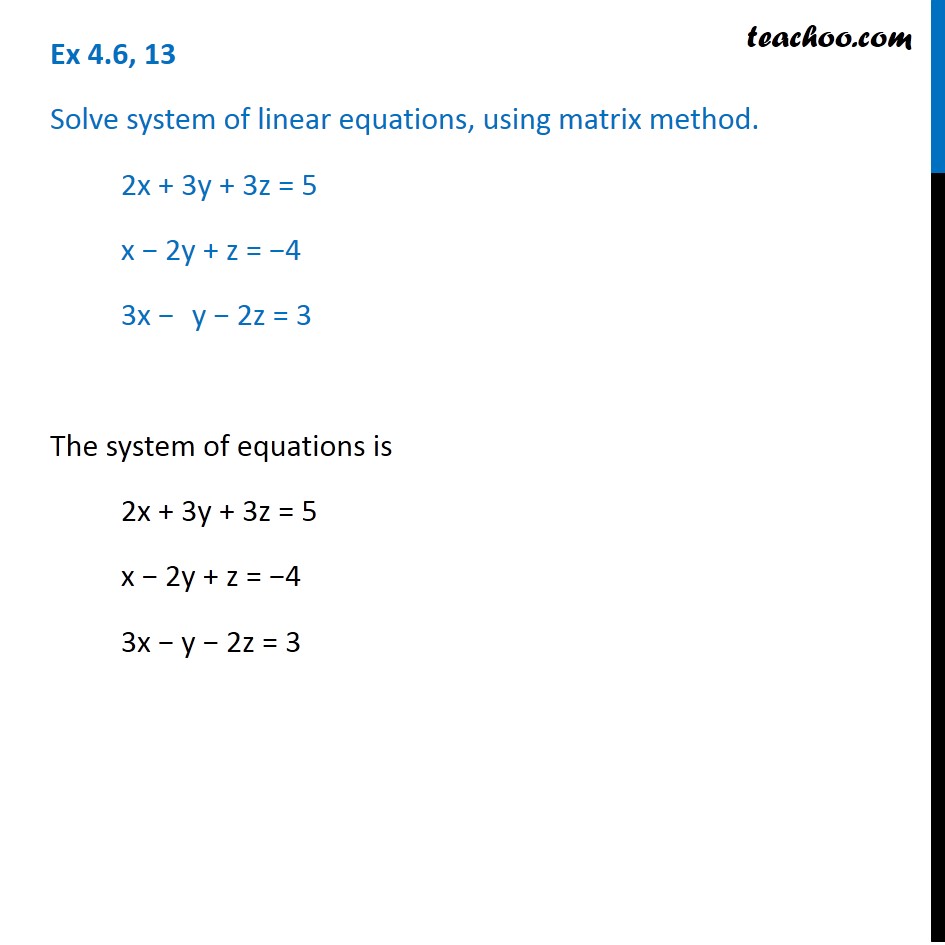
Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6

Solving Algebraic Equation Notes

Chapter 3 System Of Linear Equations 3 1 Linear Equations In Two Variables Forms Of Linear Equation Forms Of Linear Equation Ax By C A B C Are Ppt Download
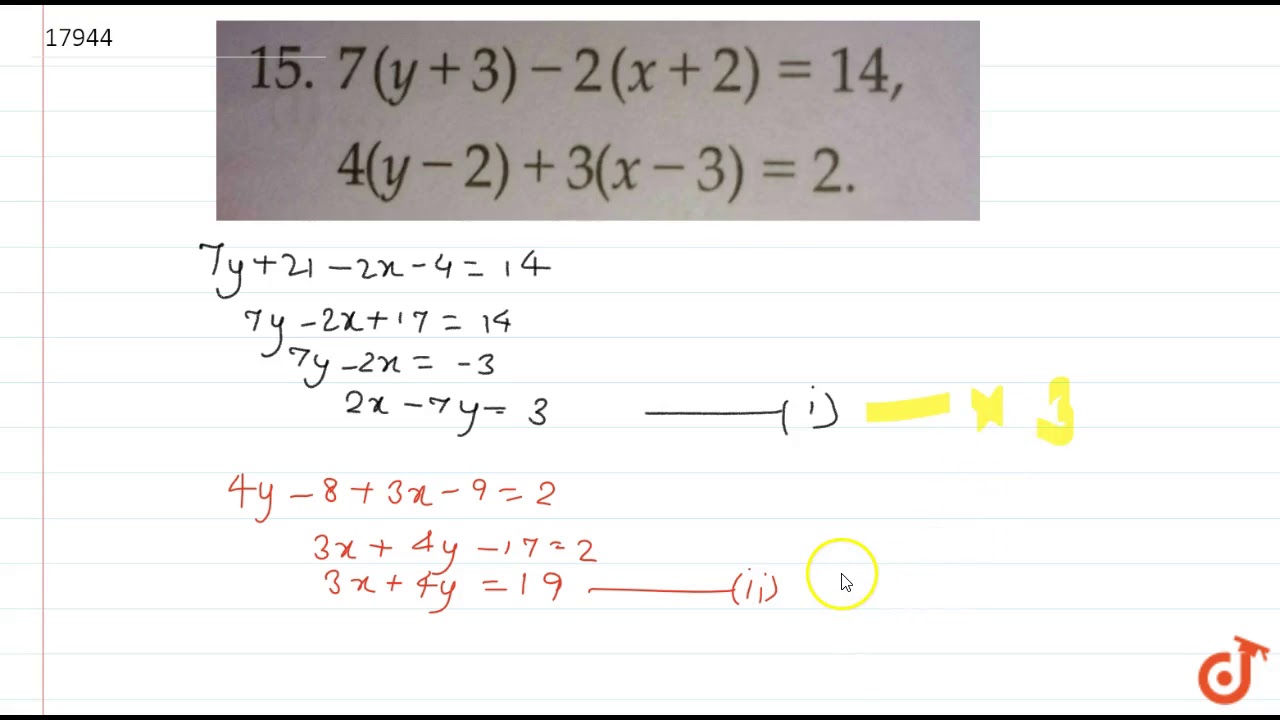
7 Y 3 2 X 2 14 4 Y 2 3 X 3 2 Youtube
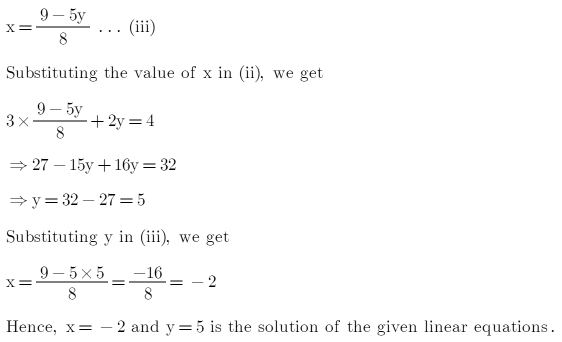
By The Substitution Method Solve The Linear Equation That Is 8 X 5 Y Is Equal To 9 And 3 X 2 Y Is Equal To 4 Mathematics Topperlearning Com Usvpr6ll




